


Next: 5 Quantum Efficiency Measurements
Up: 4 Spectral Response Function
Previous: 4.1 Objectives and Assumptions
In this section we present sample results from preliminary analyses of
spectral response function measurements made to date.
The most fundamental parameters of the spectral response function are its first
two moments (energy scale and spectral resolution), and we
confine our discussion here to these characteristics. Results
for one flight-quality device are shown in Figures 4 and 5. These data were obtained using the source described in these
proceedings by Jones et al. [5] Measurements energy scale and
spectral resolution are also made at lower energies as part of the ACIS CCD
calibration. Results of these measurements are not reported here, but
are discussed by Prigozhin et al. [6]
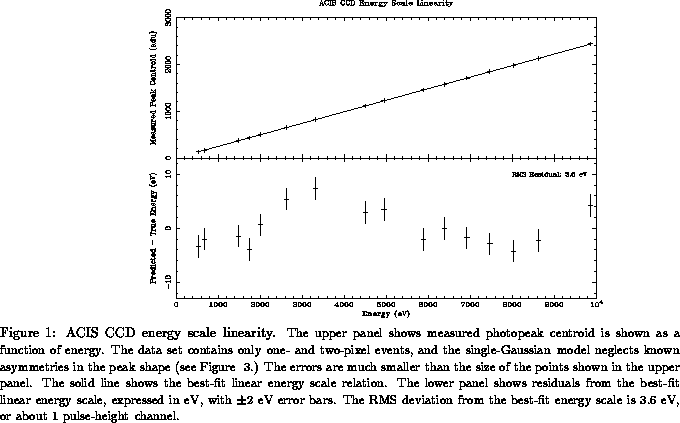
The energy scale measurements shown in Figure 4 indicate that,
at energies between 500 eV and 10 keV, a linear energy scale is remarkably
accurate: the RMS residual from the best-fit linear model is 3.6 eV.
Note that one- and two-pixel events were selected in analyzing these data.
The error bars shown on the residuals in the lower
panel of the figure, at 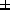 2 eV, are far larger than the statistical
precision of the peak centroid determinations. We believe that these error
bars are a conservative estimate of the systematic errors inherent in the use
of a simple, single-Gaussian model of the photo peak. Given these errors,
the formal, 90% confidence error limits for the slope are
2 eV, are far larger than the statistical
precision of the peak centroid determinations. We believe that these error
bars are a conservative estimate of the systematic errors inherent in the use
of a simple, single-Gaussian model of the photo peak. Given these errors,
the formal, 90% confidence error limits for the slope are 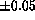 % of
the mean slope.
% of
the mean slope.
The residuals exhibit a systematic trend with energy which remains to be
investigated. A number of processes are expected to contribute to
non-linearity in the CCD energy scale. These include the variation
of charge collection efficiency with X-ray energy, and, especially at low energies, the variation
of the mean ionization energy. [12] Qualitatively, the former
effect contributes to negative residuals (in Figure 4)
at high energies, while the latter effect may contribute to negative residuals
at low energies. [12,13,14] We expect that accurate modelling of
these effects, particularly with reference to charge collection efficiency,
will improve our knowledge of the ACIS energy scale.
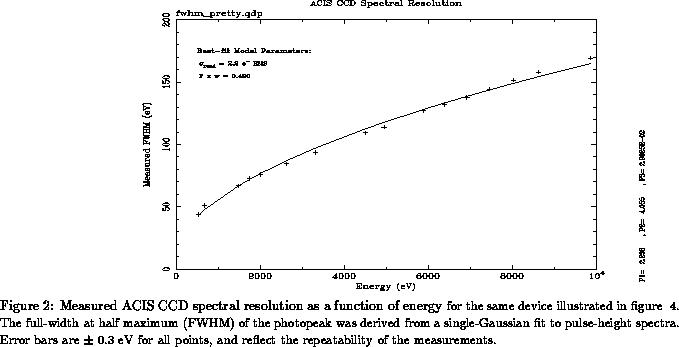
Figure 5 shows measured spectral resolution, expressed as
full-width-at-half-maximum (FWHM) of the photopeak, for the same device, and the
same data selection criteria, used for the measurements
shown in Figure 4. A simple model of the form
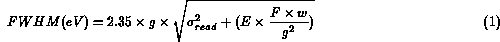
was fit to the data. Here g, the slope of the energy scale, in eV/adu,
is a fixed parameter determined from the data in Figure 4,
and E is the photon energy, in eV, F is the Fano factor and w is
the mean ionization potential per electron-hole pair. (An ``adu'' is an
analog-to-digital engineering unit for the energy scale.)
The parameters varied in the fit are
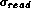 , the RMS system noise, expressed in adu, and the ratio
, the RMS system noise, expressed in adu, and the ratio
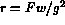 .
.
The best fit values for
the model parameters are indicated in Figure 5.
The best-fit
value for the system noise is the equivalent of 2.4 electrons, RMS, which
is consistent with the source-free measurements of this parameter. The
best-fit value 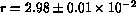 (90% confidence error
limits) implies, for example, that if
(90% confidence error
limits) implies, for example, that if 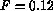 , then
, then 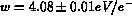 ,
which is significantly higher than the value
,
which is significantly higher than the value 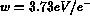 reported by
Lechner and Strueder. [12] On the other hand, the RMS residual deviation
of the model from the data is 2.5 eV, which is much larger than errors due
to counting statistics, which are expected to be about 0.3 eV RMS. Moreover,
Figure 5 clearly shows that the residuals vary systematically
with energy. These systematics must be understood before these data
can support conclusions about fundamental physical parameters.
reported by
Lechner and Strueder. [12] On the other hand, the RMS residual deviation
of the model from the data is 2.5 eV, which is much larger than errors due
to counting statistics, which are expected to be about 0.3 eV RMS. Moreover,
Figure 5 clearly shows that the residuals vary systematically
with energy. These systematics must be understood before these data
can support conclusions about fundamental physical parameters.
A number of processes must be accounted for to provide a more accurate
prediction of ACIS spectral resolution as a function of energy. Probably
the most important among these is the effect of incomplete charge collection
on the shape and width of the photopeak. It is interesting to note that,
at these very low system noise levels, the additional read-noise penalty
incurred in reconstructing multiple pixel events is not a large
contributor to spectral resolution. This is so because at low energies,
where the multi-pixel noise penalty would significantly affect resolution,
the proportion of multi-pixel events is quite low. At higher energies,
the stochastic fluctuations in the photo-ionization process dominate the
spectral resolution. In fact, for ACIS detectors operated with ACIS
electronics, the resolution penalty from reconstruction
of two-pixel events is less than 1%, in the FWHM, at all energies in the
AXAF band. Partly for this reason, the naive model
presented here predicts ACIS spectral resolution with an accuracy of
order 5% in the FWHM.



Next: 5 Quantum Efficiency Measurements
Up: 4 Spectral Response Function
Previous: 4.1 Objectives and Assumptions
mwb@space.mit.edu
 2 eV, are far larger than the statistical
precision of the peak centroid determinations. We believe that these error
bars are a conservative estimate of the systematic errors inherent in the use
of a simple, single-Gaussian model of the photo peak. Given these errors,
the formal, 90% confidence error limits for the slope are
2 eV, are far larger than the statistical
precision of the peak centroid determinations. We believe that these error
bars are a conservative estimate of the systematic errors inherent in the use
of a simple, single-Gaussian model of the photo peak. Given these errors,
the formal, 90% confidence error limits for the slope are 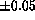 % of
the mean slope.
% of
the mean slope.
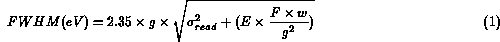
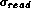 , the RMS system noise, expressed in adu, and the ratio
, the RMS system noise, expressed in adu, and the ratio
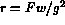 .
.
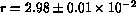 (90% confidence error
limits) implies, for example, that if
(90% confidence error
limits) implies, for example, that if 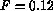 , then
, then 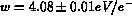 ,
which is significantly higher than the value
,
which is significantly higher than the value 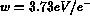 reported by
Lechner and Strueder. [
reported by
Lechner and Strueder. [
